Doing Physics with Quaternions

Video Ed

Technical shows, Not-so-technical, quaternion animations, all served up from YouTube. Watch and learn!

Visual Physics.org

A community research web site centered on visualing quaternions, often motivated by physics.

Math (pdf)

An overview

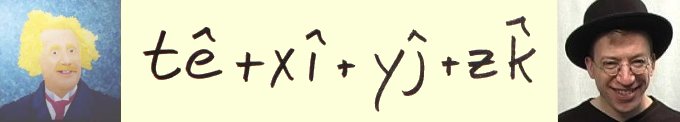
A brief history of quaternions

Multiplying quaternions the easy way

Inner and outer products

Scalars, vectors, tensors and all that

Quaternion analysis (pdf, related video: "Why Quantum Mechanics is Weird")

Topology

Where quaternions fit in math, adapted from Max Tegmark, 1998.

Tools for algebra, trig, logs...

Java Quaternion calculator

- written in Java 1.0, so is broken :-(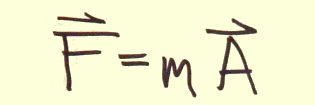

Dynamic graphs

Physics

Classical Physics (pdf)

F = m a, in an inertial frame, in polar coordinates, and in a rotating reference frame

The simple harmonic oscillator and wave equation

4 tests for a conservative force

Unifying Gravity and EM

I am rescinding my efforts to unify gravity and EM using quaternions and hypercomplex numbers. The quaternions do the work of EM fine and that part of this work is still of value. The hypercomplex numbers were given the chore of doing gravity. Under a rotation, the square of a hypercomplex number changes. As such, when used in a Lagrangian, angular momentum will not be conserved. Gravity most definitely conserves angular momentum. There may be things people can learn (how to derive field equations using Lagrange densities, the precession of the perihelion of Mercury, ...).

Simply put, all of the work, claims, and even the t-shirt, that touch on hypercomplex numbers are WRONG. I learned a huge amount of real physics anyway. You might also. Good luck in your studies of this flawed work.

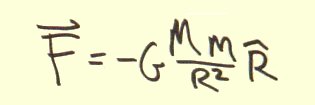
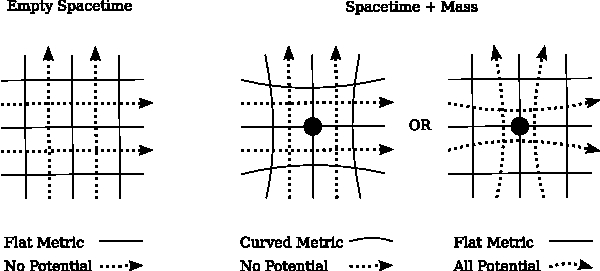
I think it is reasonable to believe I have found a way to unify gravity with the forces of electromagnetism, the weak and strong forces in a way that can been quantized. Only unloved math tools are used. The problem is a puzzle to be solved, not a math problem so difficult only a few folks at the Institute for Advanced Study to understand. At the core is the action, shown here, which requires both quaternions and hypercomplex multiplication (indicated by the box-times), keeping only the scalar. My paper is here ( Mathematica notebook, html, or pdf)

Brief summaries or calculations

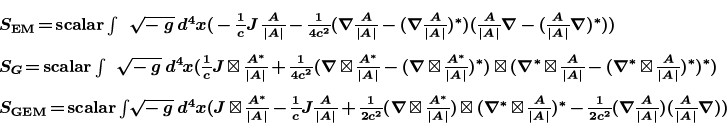
The Unified GEM Action and Symmetries (pdf)

The Unified GEM Lagrangian, Field Equations, and Solutions (pdf)

The Precession of the Perihelion of Mercury Based on the Unifed GEM Proposal (pdf)

Derivation of the GEM unified field proposal using quaternions exclusively (pdf)

Slides from talks

Geometry + 4-Potentials = Unified Filed Theory, 2007 April APS talk available as html or YouTube video.

Unifying Gravity and EM, a Riddle You Can Solve (pdf), 9th Eastern Gravity Meeting, 2006.

Why a Rank 1 Unified Field Theory is Compelling and Its Background Mathematical Structure (pdf), 8th Eastern Gravity Meeting, 2005.

Unifying Gravity and EM by Generalizing EM (pdf), 7th Eastern Gravity Meeting, 2004.

Unifying gravity and EM by analogies with EM, MIT IAP course, Jan. 2003.

Lagrange Densities (pdf, video 1, video 2)
Fields and Quantum Mechanics (pdf, video 3, video 4)
Forces, Metrics, and New Physics (pdf, video 5, video 6)

Realizing Einstein's Dreams: Unifying Gravity and Light, and Why Quantum Mechanics is Weird (pdf), Joint APS/AAPT meeting, Spring 2005.

4-potential Equations for Gravity and Electromagnetism (pdf), Joint APS/AAPT meeting, Fall, 2003.

The Cosmological Consequences of the Product Rule (pdf), Joint APS/AAPT meeting, Spring, 2004. A Universe with zero dark matter is good :-)

Picture Stories of Events in Spactime (pdf), Do While Studios, Spring 2000. The relationship between Special and General relativity using quaternions.

Papers

A family of variations on the Maxwell action using quaternions and hypercomplex numbers contains the symmetries of the standard model and a testable gravity theory (pdf)
(my current best effort, version 20091119)

OLDER EFFORTS... an approaching using standard tensors and differential geometry

Deducing a Unified Field Theory from Electromagnetism (pdf).

Unifying Gravity and EM by analogies to EM (pdf) (v 4.4, 13 pages).

Geometry + 4-Potential = Unified Field Theory, submission for the 2007 Essays on Gravitation, sponsored by the Gravity Research foundation (pdf)

An approach using quaternions.

Einstein's Vision I: Classical unification of gravity and electromagnetism using Riemannian quaternions (pdf)

Einstein's Vision II: Unified force with constant velocity profiles (pdf).

Ropes and Gravity

Posters

Unifying Gravity and EM by analogies to EM (pdf) Brookhaven National Lab meeting.

Mathematica notebooks

From the Lagrange density to experimental tests (pdf, or .nb)

Videos!

will have all my latest video-education efforts. Enjoy.

--boxed

Electromagnetism (pdf)

Classical electrodynamics

Electromagnetic field gauges

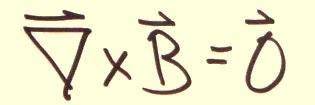
The Maxwell equations in the light gauge: QED?

The Lorentz force

The stress-energy-momentum 2-tensor of the electromagnetic field

Paper: Maxwell's vision: electromagnetism with Hamilton's quaternions (pdf), Second Meeting on Quaternionic Structures in Mathematics and Physics, Rome, 1999.