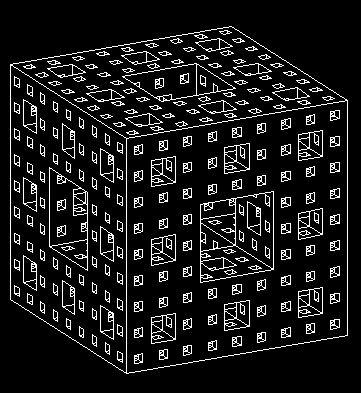

Menger's sponge (sometimes wrongly called Sierpinski's Sponge) is a fractal solid that can be described as follows. Take a cube, divide it into 27 = 3 x 3 x 3 smaller cubes of the same size and remove the cube in the center and the six cubes that share faces with it. You are left with the eight small corner cubes and twelve small edge cubes holding them together. Now, imagine repeating this process on each of the remaining 20 cubes. Repeat again. And again ...
Menger's Sponge is named for its inventor, Austrian mathematician Karl Menger (1902-1985). For more information about fractals and Menger's Sponge, visit the following web site:
Self Similar Fractals
Fractals - Fractal Dimension
or look for "The Fractal Geometry of Nature" by B. Mandelbrot (W.H.Freeman 1982) in your local bookstore or library.